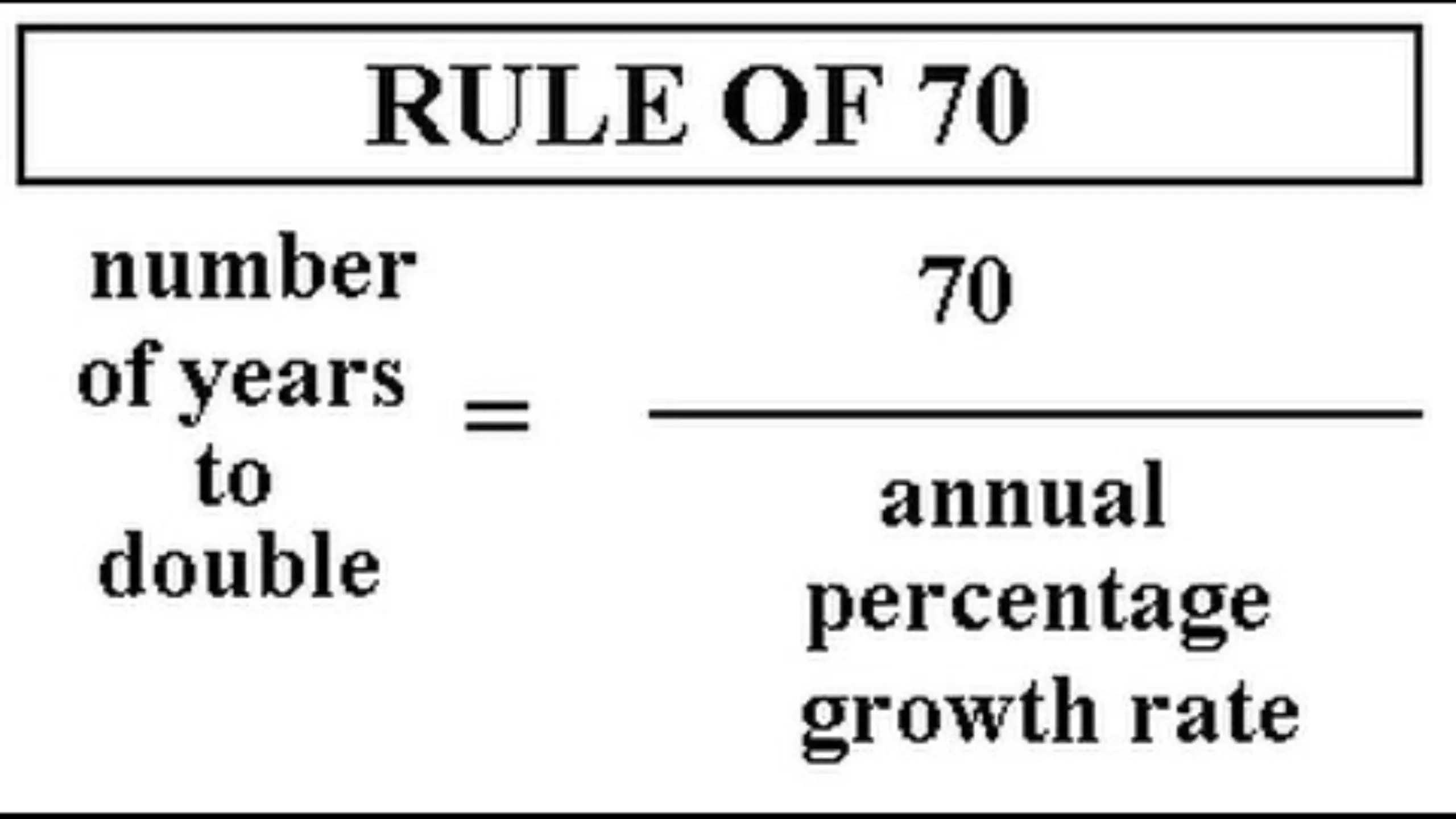
The Rule of 70 is a popular tool used in finance, economics, and other fields to estimate time. It takes a quantity to double based on its compounded annual growth rate (CAGR). This rule is widely used because it is simple and intuitive, and provides reasonably accurate estimates for a wide range of growth rates. This article will explain the concept of the Rule of 70, give some examples of its application, and discuss its limitations and alternatives.
The Mathematics Behind the Rule of 70
The Rule of 70 is based on the logarithmic function, which describes the relationship between the growth rate of a quantity and the time it takes to double. Specifically, if we denote the growth rate as r (expressed as a decimal), the time it takes to double as t. The initial quantity is Q, then we have the following equation:
Q x (1 + r)^t = 2Q
Solving for t, we get:
t = ln(2) / ln(1 + r)
Using the fact that ln(1 + r) ≈ r for small values of r, we can simplify the equation as:
t ≈ ln(2) / r
This is where the Rule of 70 comes in. We can approximate ln(2) as 0.693, and thus we have:
t ≈ 0.693 / r
Since 0.693 is close to 70, we can use this number instead of ln(2) to simplify the calculation:
t ≈ 70 / r
This is the Rule of 70 in its simplest form: to estimate the time it takes for a quantity to double, divide 70 by the compounded annual growth rate.
Examples of the Rule of 70 in Practice
The Rule of 70 can be applied to a wide range of scenarios where compounded growth rates are relevant. Some examples include:
- Investing: If an investment has a CAGR of 7%, it will take approximately 10 years for the investment to double in value (70/7).
- Population growth: If a country’s population is growing at a CAGR of 2%. It will take approximately 35 years for the number of people to double (70/2).
- Inflation: If the inflation rate is 3%, prices will double in approximately 23 years (70/3).
- Debt: If the interest rate on a loan is 5%, the amount owed will double in approximately 14 years (70/5).
Limitations and Alternatives of the Rule of 70
While the Rule of 70 is a useful tool for estimating growth rates, it has some limitations that should be taken into account:
- It assumes a constant growth rate:
The Rule of 70 assumes that the growth rate is constant over time, which may not always be the case. In reality, growth rates may fluctuate due to various factors such as economic cycles, policy changes, and technological innovations.
- It is not precise for small or large growth rates:
The Rule of 70 provides reasonably accurate estimates for growth rates between 2% and 10% but becomes less accurate for smaller or larger rates. For example, if the growth rate is 1%, it will take approximately 70 years to double. Which is much longer than the estimate of 35 years provided by the Rule of 70.
- It does not account for compounding periods:
The Rule of 70 assumes annual compounding, but in some cases, the compounding may occur more frequently, such as quarterly or monthly.
To overcome these limitations, there are alternative methods that can be used to estimate growth rates. Such as the Rule of 72, which is similar to the Rule of 70 but uses the number 72 instead of 70. The Rule of 72 is more accurate for smaller growth rates and can be adjusted for different compounding periods by dividing. The interest rate by the number of compounding periods per year.
Another alternative is to use a spreadsheet or financial calculator to calculate the exact time. It takes a quantity to double based on the specific growth rate and compounding period. This method provides more precise estimates but requires more complex calculations.
Here are some details on the Rule of 70 and its applications:
- The Rule of 70 is based on the exponential function
- The Rule of 70 can be used to estimate not only the time
- The Rule of 70 can be applied to both positive and negative growth rates.
- The Rule of 70 can also be used to compare the growth rates of different quantities over the same period.
- The Rule of 70 can be useful for long-term planning and forecasting
In The End:
In conclusion, the Rule of 70 is a simple yet powerful tool for estimating compounded growth rates and the time it takes for a quantity to double. While it has some limitations, it can provide reasonably accurate estimates for a wide range of growth rates and can be applied to various fields and scenarios. By using the Rule of 70 as a quick estimation tool and understanding. With its underlying mathematics, individuals, and organizations can make more informed decisions about their finances, investments, and plans.